Tag Archives: Volume

346. Area & Volume conversion

Leave a reply

This is a quick post on how I teach metric unit conversion for area and volume. All you need is a big whiteboard and coloured board pens.

Start by stressing that all diagrams are not to scale/accurate.

Two colours

  1. Draw a square on the board
  2. Pen colour 1: Label it as 1cm
  3. What is the area? Show the calculation
  4. What is 1cm in mm?
  5. Pen colour 2: Label it as 10mm
  6. What is the area in mm? Show the calculation
  7. What is the scale factor between the sides? the area? why?


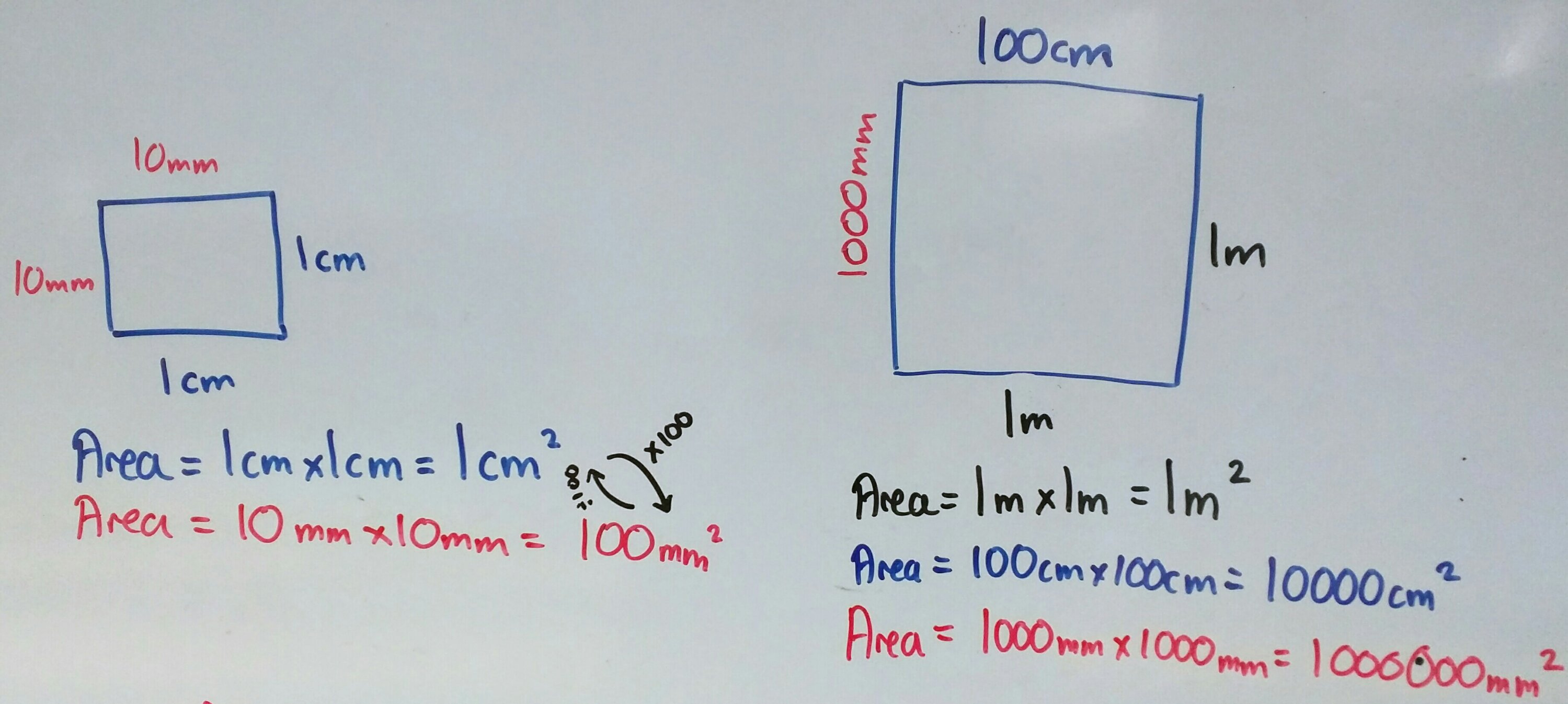
Three colours

  1. Draw a square on the board
  2. Pen colour 3: Label it as 1m
  3. What is the area? Show the calculation
  4. What is 1m in cm?
  5. Pen colour 2: Label it as 100cm
  6. What is the area in cm? Show the calculation
  7. What is the scale factor between the sides? the area? why?
  8. Repeat in pen colour 1 for mm

Four colours

Well not actually four colours – pens 2,3 & 4 only. Repeat the process for kilometres to metres and centimetres.

Volume – same process, just three dimensions

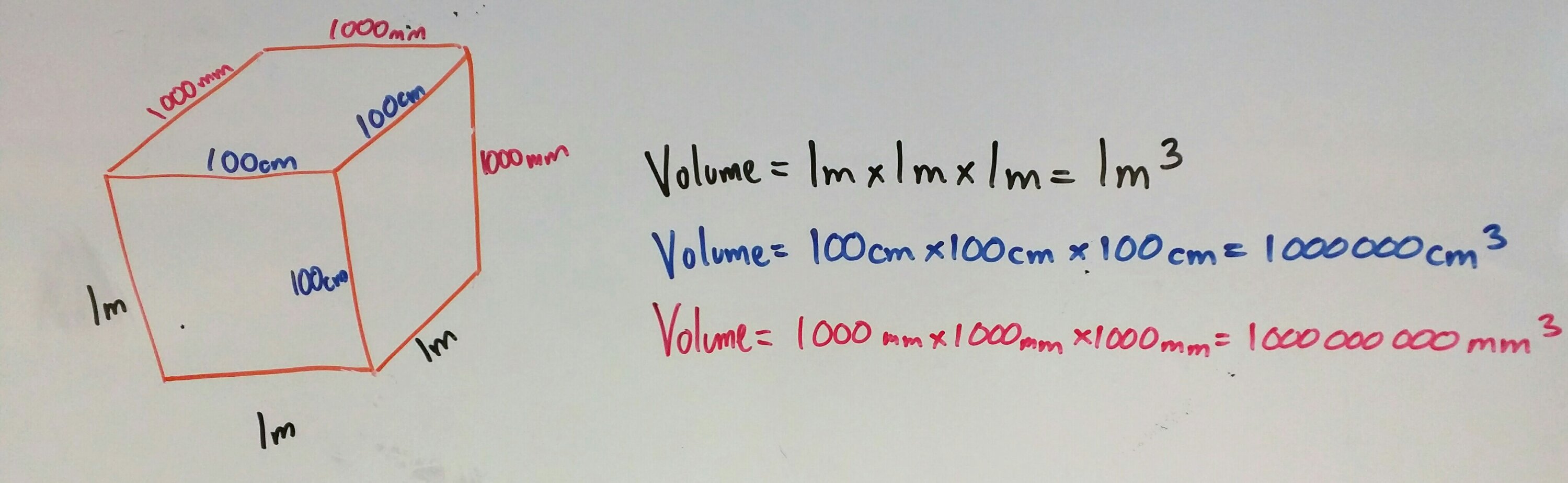
Why all the colours?

By coding each unit of measurement with a colour students can see the progression of the calculations and the links between area/volume and scale factor. After all, an okay mathematician can reproduce memorised facts, but a great mathematician doesn’t need to memorise – they understand where the calculations came from.

293. Boxing Bounds

Leave a reply

I thought this would make a nice little starter – address a few different topics, bit of problem solving, all over in 15 minutes. How wrong I was!

The Question: A company packs toys into boxes which measure 12cm by 8cm by 10cm (to the nearest centimetre). The boxes are packed into crates which measure 1m by 0.75m by 0.8m (to the nearest centimetre).
(a) Basic question – How many boxes fit into the crate?
(b) What is the maximum volume of a toy box?
(c) What is the minimum volume of the crate?
(d) Look at your answers to (b) and (c) – do they affect your answer to (a)?

It was a simple question about fitting toy boxes into a shipping crate. It extended to looking at upper and lower bounds, then recalculating given this extra information. Simple? No chance!

Problem One
Not changing to the same units

Problem Two
Working out the two volumes and dividing to find the number of toys. When challenged on this, it took a while to get through to the basics of how many toys actually fit – mangled toys and split up boxes don’t sell well.

Problem Three
Maximising the arrangement of boxes – remainders mean empty space

Problem Four
Using the information from Problem Three to find the total number of toys

Problem Five
Working out the dimensions and volume of the empty space in the box

Problem Six
Trying to convert centimetres cubed into metres cubed. I don’t even know why they wanted too!

Problem Seven/Eight
What’s an upper/lower bound?

Problem Nine
What do you mean that the original answer changes when the box size alters?

Problem Ten
All those who weren’t paying attention when you went over Problem Two and don’t ‘get’ why the answer isn’t 625!

245. Fair share

Leave a reply

I spotted this ‘Expert Tip’ whilst flicking through a supermarket magazine:

image

Image credit: tesco.com/foodandliving

Question
If this cake has a diameter of 18cm (7in), is this a fair way to split it between guests? Can you prove your result in general terms?

Of course, this assumes that the icing on the side doesn’t count in the diameter or guest preference.

234. (Students) cubed

1 Reply

Here is a quick fun starter to get your class thinking about dimensions and volume.

Question: How many students can you fit into a metre cube?

The discussion will probably include:

  • ‘How big is a metre?’ (find a metre stick)
  • ‘Did you mean standing in a square?’ (no)
  • ‘How big is the student?’ (average – that answer annoys students)
  • And finally ‘Huh?’ (ask the person next to you to explain)

Whilst this is going on make a metre square on the wall and the floor, using duct tape. The inner measurements of the cube are 1m, the rest is just tape border.

image

The Predictions
Draw up a quick tally chart of how many students they think will fit. A bright child will usually ask how are you going to find out. Easy …

Put students in the cube
Let them put themselves into the confines of the cube. Cue bouncy boys squashing up. Then remind them it can’t be higher than a metre. You might find it useful to have two spare students hold metre sticks vertically at the non-wall end to define the end of the cube.

We managed nine boys, plus gaps at the top for bits of a tenth boy – it wasn’t ethical to chop one up and sprinkle the bits. So we imagined the tenth person balanced on the gaps around their shoulders.

Ten? That is a new record for this activity!

image

The Point

  • Volume is the space inside a 3D shape.
  • One metre cube is bigger than you think.
  • It’s a memorable activity to refer back to.

Even better if …

I’d love to get sturdy board covered in birthday (or Christmas) paper to put under and around the cube to start a discussion about surface area. You could make a big show of unfolding the cube and laying the wrapping out on the floor to form a huge net.

 

Note
I used to do this by taping metre sticks into a cube, but they fell apart easily. In some schools three metre sticks is a challenge, twelve would be a miracle find. Duct tape works much better!

232. Steps in Volume

2 Replies

This is a quick little post to give you a nifty little resource inspired by the ideas of Bruno Reddy (@MrReddyMaths). I suggest you visit his website at: http://mrreddy.com/.

Sphere cone pyramid

Image Credit: http://k12math.com/math-concepts/algebra/volumes/volumes.htm

I’ve been teaching my class how to calculate the volume of spheres, cones and pyramids. They really like these staged worksheets. You could print them out as they are, but I personally print them as A5 booklets which fit into their books.

Volume of Sphere Cone Cylinder (pdf)

204. Revolution in Volume

Leave a reply

Most elements of Core Maths can be visualised with a good diagram, but volume of revolution can be tricky if your technical drawing skills leave something to be desired. My colleague JA came up with a visualisation which is simple and elegant, yet also fun and memorable.

Step 1
Start with a curve. Introduce the limits a and b. Discuss what shape a thin strip would make: a disc.

image

Step 2
What would several discs make?
Now this is the cool bit:

image

This innocent looking shape is a pop up gift tag:

image

You can demonstrate what happens if the curve rotates 180 degrees around the x-axis.

Step 3
Now the really fun bit: dig out those interesting honeycomb christmas decorations, a metre stick and some tape:
image

The metre stick represents the scale on the x axis. The decoration represents the full 360 degree revolution about the axis.

Hint
Since these decorations are made from paper and card. You can use a sturdy craft knife to cut them into other curves. They also make great wall displays.